Domain Mathematical Definition

In mathematical analysis a domain is any connected open subset of a finite dimensional vector space.

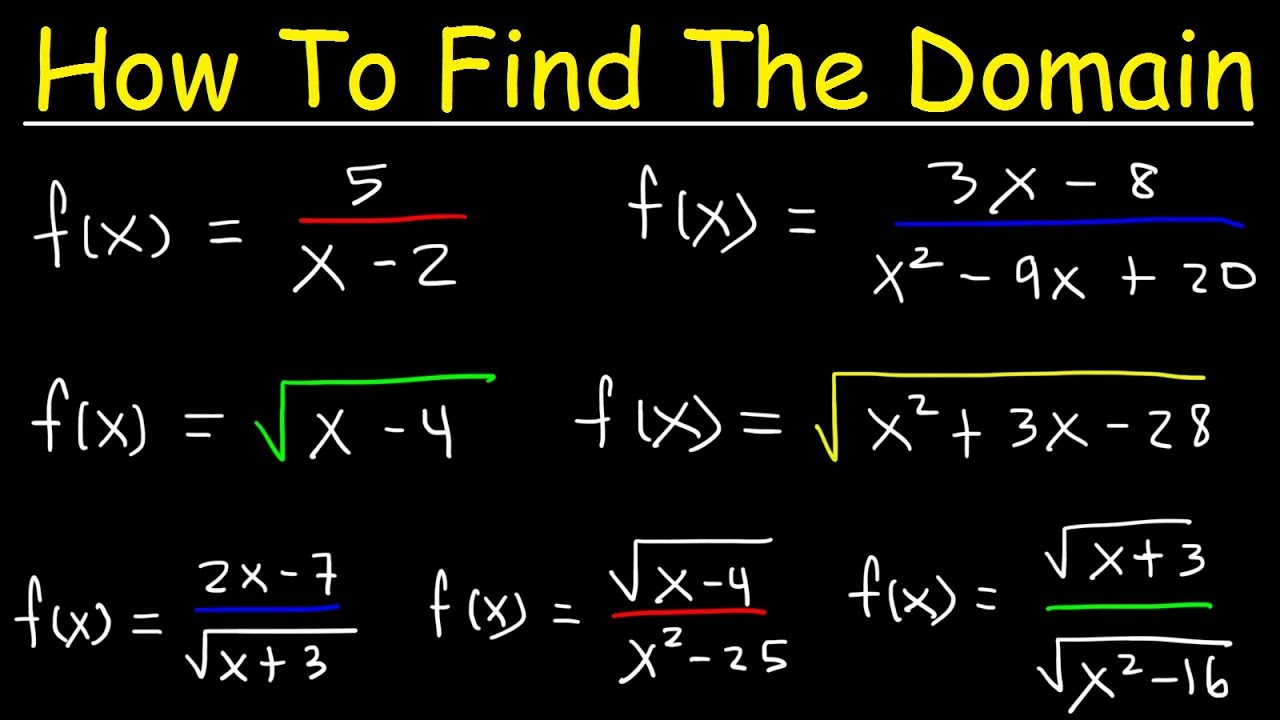
Domain mathematical definition. In mathematics the domain or set of departure of a function is the set into which all of the input of the function is constrained to fall. Domain the set of values of the independent variable s for which a function or relation is defined. A simple mathematical function has a domain of all real numbers because there isn t a number that can be put into the function and not work. The domain is the set of all possible x values which will make the function work and will output real y values.

All the values that go into a function. Domain in math is defined as the set of all possible values that can be used as input values in a function. However this coincidence is no longer true for a partial function since the domain of definition of a partial function can be a. Math mathematics maths a science or group of related sciences dealing with the logic of quantity and shape and arrangement.

X y and is alternatively denoted as dom displaystyle operatorname dom. It is quite common for the domain to be the set of all real numbers since many mathematical functions can accept any input. Domain of a function mathematics the set of values of the independent variable for which a function is defined. The set of all possible input values commonly the x variable which produce a valid output from a particular function.

Is defined for all real values of x because there are no restrictions on the value of x. Since x 2 is never negative x 2 2 is never less than 2 hence the range of f x is all real numbers f x 2. Domain function range. Since a function is defined on its entire domain its domain coincides with its domain of definition.

A mathematical relation such that each element of the input is paired with exactly one output. The output values are called the range. Hence the domain of f x is all real values of x. F x x 2 2.

This is a different concept than the domain of a function though it is often used for that purpose for example in partial differential equations and sobolev spaces. Typically this is the set of x values that give rise to real y values. When the function f x x2 is given the values x 1 2 3 then the domain is simply those values 1 2 3 domain range and codomain.