Inverse Of Diagonal Matrix Math

Since every submatrix has 1 on the diagonal and zero otherwise the matrix itself has 1 on the diagonal and zero otherwise so that.

Inverse of diagonal matrix math. Pivot on matrix elements in positions 1 1 2 2 3 3 continuing through n n in that order with the goal of creating a copy of the identity matrix i n in the left portion of the augmented matrix. Dn 1 d 1 1 0. Displaystyle forall i j in 1 2 ldots n i neq j implies d i j 0. If all the numbers in the matrix are 0 except for the diagonal numbers from the upper right corner to the lower left corner it is called an anti diagonal matrix.

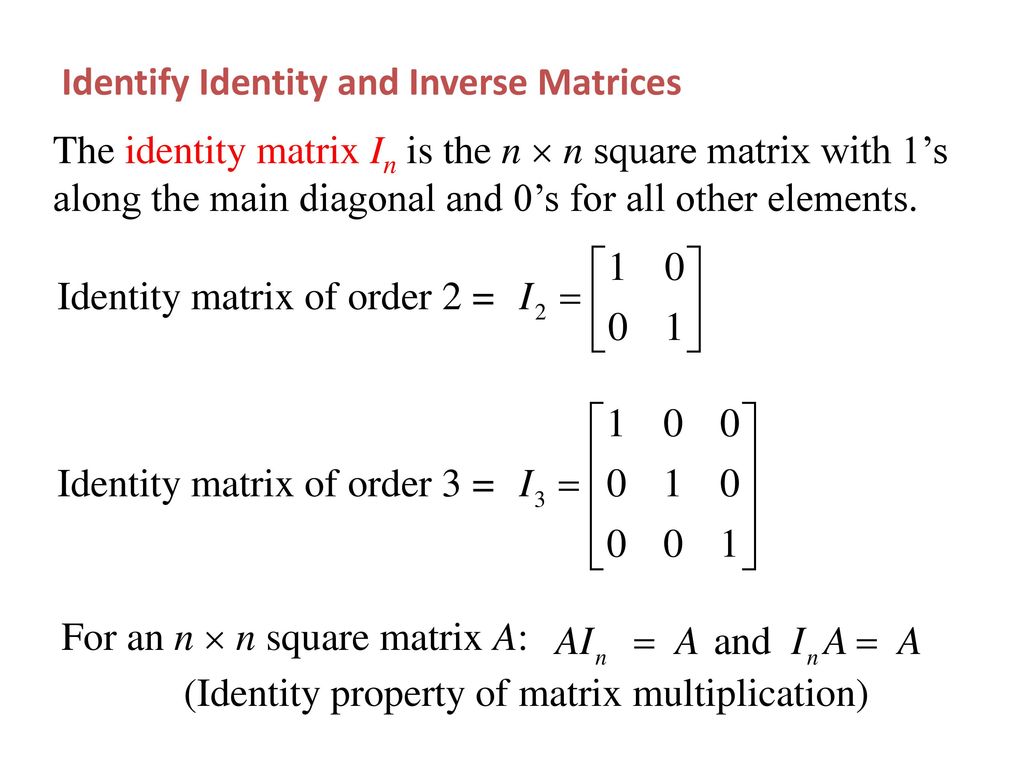
Inverting diagonal matrices theorem a diagonal matrix d diag d1 dn is invertible if and only if all diagonal entries are nonzero. When we multiply a matrix by its inverse we get the identity matrix which is like 1 for matrices. 1 therefore to form the inverse of a diagonal matrix we will take the reciprocals of the entries in the main diagonal. Same thing when the inverse comes first.

8 1 8 1. That is the matrix d di j with n columns and n rows is diagonal if. It is represented as. This diagonal matrix is invertible if all of the entries on the main diagonal are nonzero that is for every.

When we multiply a number by its reciprocal we get 1. It fails the test in note 5 because ad bc equals 2 2 d 0. For example consider the following diagonal matrix. If no such interchange produces a non zero pivot element then the matrix a has no inverse.

The matrix is therefore a right left inverse for. A square matrix d d ij n x n will be called a diagonal matrix if d ij 0 whenever i is not equal to j. Since is both a left and a right inverse for is therefore the inverse of. From the way was constructed we then have.

Di 6 0 for 1 i n. The inverse of a diagonal matrix. A square matrix in which every element except the principal diagonal elements is zero is called a diagonal matrix. As stated above a diagonal matrix is a matrix in which all off diagonal entries are zero.

Properties of diagonal matrix. If a d 2 6 4 d1 dn 3 7 5 then a 1 d 2 6 4 1 d1 1 dn 3 7 5. A 1 a i. Solving linear equations note 6 a diagonal matrix has an inverse provided no diagonal entries are zero.

The determinants of the above matrix are d x 11 x 22 x 33 anti diagonal matrix. If one of the pivoting elements is zero then first interchange it s row with a lower row. A a 1 i. Example 1 the 2 by 2 matrix a d 12 12 is not invertible.

If d is invertible then d 1 diag d 1 1 d 1 n. 1 8 8 1. Let us consider a diagonal matrix. 0 0 d 1 2.