Recursive Function In Discrete Mathematics Ppt

Please see the updated video at https youtu be j 7bq6v5zpothe full playlist for discrete math i rosen discrete mathematics and its applications 7e can.

Recursive function in discrete mathematics ppt. Discrete mathematics recursive de nitions 8 18. Cs 441 discrete mathematics for cs m. A recurrence relation is an equation that recursively defines a sequence where the next term is a function of the previous terms expressing fn as some combination of fi with i n. In mathematics we can create recursive functions which depend on its previous values to create new ones.

Iintuition 1 de nition of fnhas two base cases. There are certain arguments called base values for which the function does not refer to itself. F4 3 and 2. Rosen discrete mathematics and its applications 5th edition.

A function f. Chapter 6 recursive k. Ibase case 1 n 3. Example fibonacci series fn fn 1 fn 2 tower of hanoi fn 2fn 1 1.

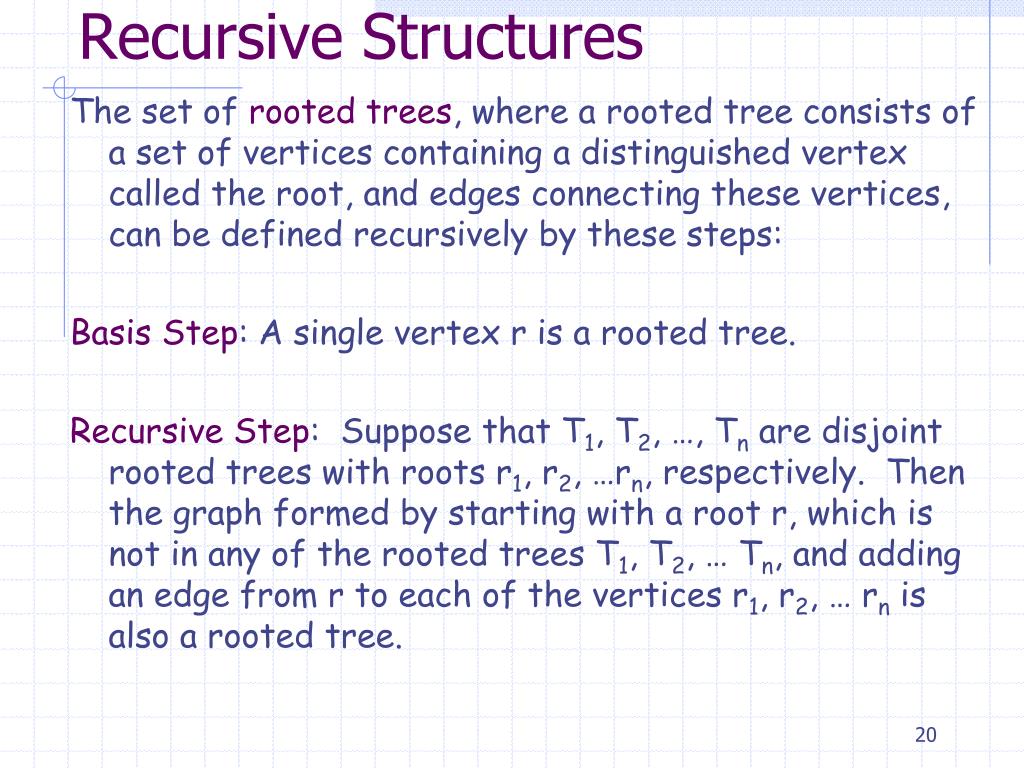
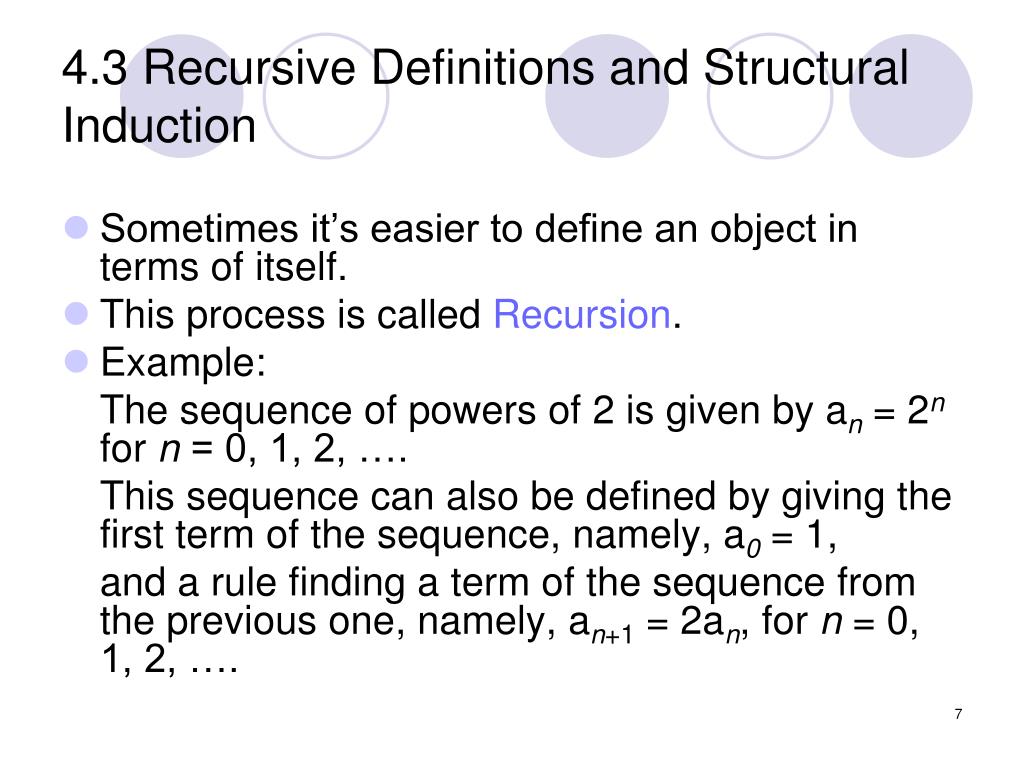
Each time the function does refer to itself the argument of the function must be closer to a base value. Iintuition 2 recursive step uses fn 1 fn 2 strong induction. In the recursive de nition of a set the rst rule is the basis of recursion the second rule gives a method to generate new element s from the elements already determined and the third rule binds or restricts the de ned set to the elements generated by the rst two rules. Recursive definitions the sequence of powers of 2 is given by a n 2 n for n 0 1 2 mldr.

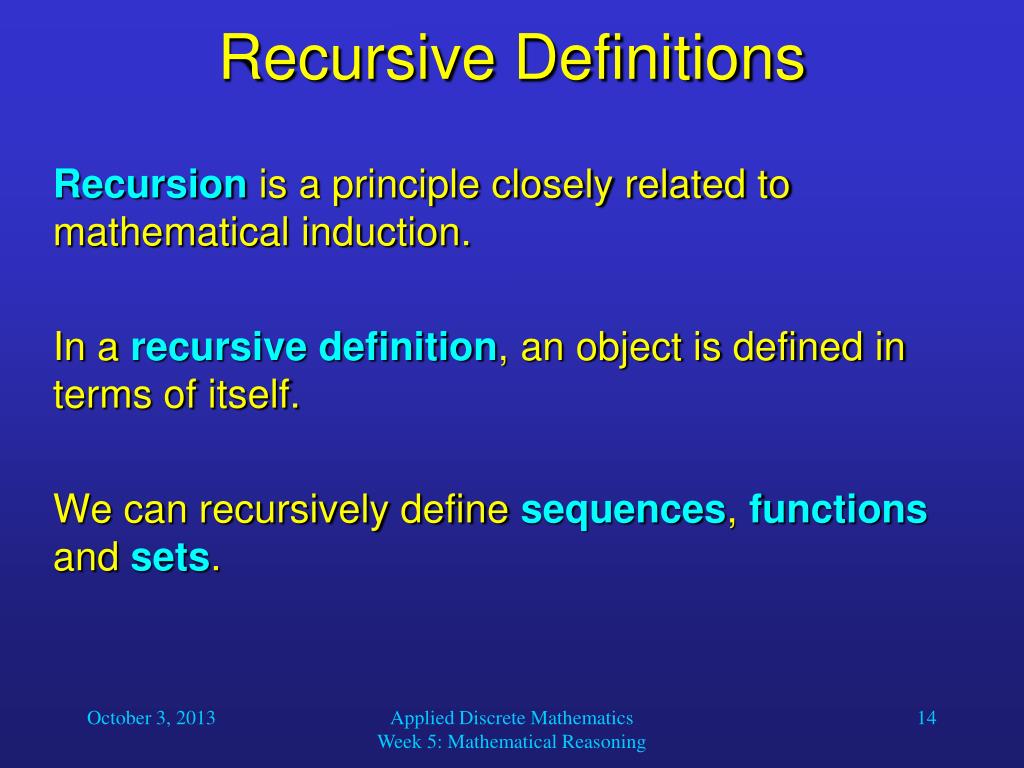
We often call these recurrence relations. Recursive step give a rule for finding its value at an integer from its values at smaller integers. This process is called recursion. Recursively defined functions definition a recursive or inductive definition of a function consists of two steps.

An a nd an an 1 d a0 a. F3 2 and 2 thus f3. Ibase case 2 n 4. Can also be defined by a 0 1 and a rule for finding a term of the sequence from the previous one i e a n 1 2a n can use induction to prove results about the sequence structural induction.

3 p 5 2 3. Hauskrecht recursive definitions sometimes it is possible to define an object function sequence algorithm structure in terms of itself. Basis step specify the value of the function at zero. For example we can have the function f x 2f x 1 with f 1 1 if we calculate some of f s values we get 1 2 4 8 16.

Is l dillig cs311h. Recursively defined functions o a function is said to be recursively defined if the function refers to itself such that 1. However this sequence of numbers should look familiar to you.